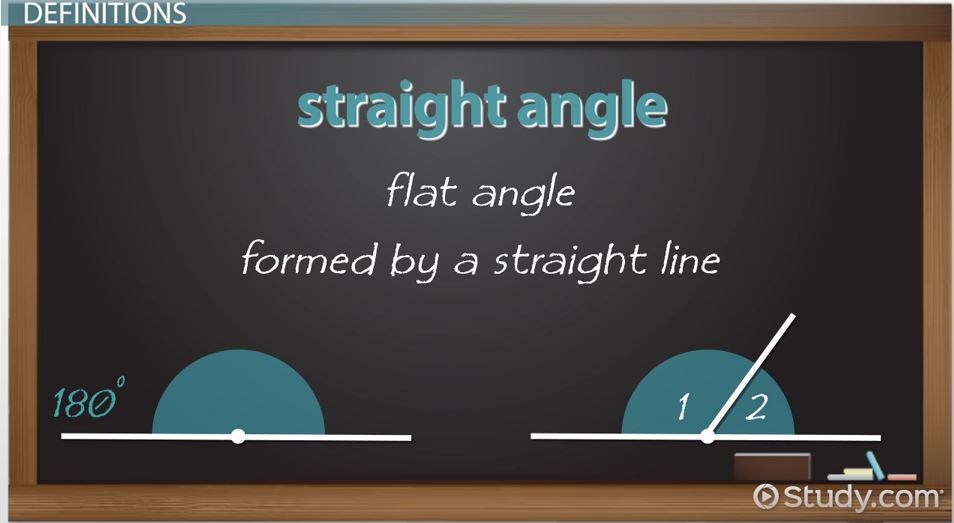
The congruent supplements theorem states that if two angles are supplements of congruent angles, then the two angles are congruent as well. This theorem is widely used in geometry proofs to establish the equality of angles.
Geometry is a branch of mathematics that focuses on the study of shapes, sizes, and positions of objects in space. One of the main concepts in geometry is the relationship between angles, which are formed by the intersection of two lines or rays.
The congruent supplements theorem is an essential principle that helps to solve complex geometric problems. This theorem states that if two angles are supplementary and congruent to other angles, then they are themselves congruent. The theorem is useful in establishing relationships between angles in different shapes such as triangles, quadrilaterals, and polygons. Geometry students use the congruent supplements theorem to prove various geometric propositions and shapes.
Credit: magoosh.com
Understanding Congruent Supplements Theorem
Congruent supplements theorem is an essential topic in geometry. It is a basic concept that plays a significant role in solving mathematical problems. Understanding this theorem is crucial, as it can help students excel in math. In this blog post, we will discuss the definition of the theorem, the formula for calculating congruent supplements, and an example problem to demonstrate the theorem.
Definition Of The Theorem
The congruent supplements theorem is a proposition that states that if two angles are supplementary to the same angle or to congruent angles, then these two angles are congruent. In simpler terms, if two angles add up to 180 degrees and they have the same or equal angles, then these two angles are congruent.
The importance of this theorem lies in its ability to simplify geometric calculations.
Formula For Calculating Congruent Supplements
To find two congruent supplements, you can use the following formula:
Angle A + Angle B = 180 Degrees
Angle C + Angle B = 180 Degrees
Angle A = Angle C
Here, a and b are supplementary angles as are c and b, and angle a is congruent to angle c. by using this formula, you can find out the value of any unknown angle in a given problem.
Example Problem To Demonstrate Theorem
To better understand the theorem, let’s consider an example problem.
Given angle a and angle b are supplementary, and angle c and angle b are supplementary. If angle a measures 60 degrees, what is the measure of angle c?
Solution:
- We know that
Angle A + Angle B = 180 Degrees
Angle C + Angle B = 180 Degrees
- We also know that angle a measures 60 degrees
- So, we can substitute value of angle a in the first equation.
60 Degrees + Angle B = 180 Degrees
Angle B = 120 Degrees
- Now, we can substitute angle b’s value in the second equation
Angle C + 120 Degrees = 180 Degrees
Angle C = 60 Degrees
- Therefore, angle c measures 60 degrees.
Congruent supplements theorem is a fundamental concept in geometry. Understanding it will help students tackle mathematical problems with ease. By keeping the formula and theorem in mind, they can easily calculate the value of unknown angles to solve geometry problems quickly and efficiently.
Properties Of Congruent Supplements Theorem
Congruent supplements theorem is crucial in geometry as it provides a significant relationship between the angles of a triangle. It states that if two angles are supplements to the same angle, then they are congruent. Properties of congruent supplements theorem are an essential aspect to analyze for clear understanding of the theorem.
Proof Of The Theorem
The theorem can be proved using simple algebraic equations. Consider two angles a and b that are supplements to another angle c. where a and b are measures of the angles in degrees. The sum of a and b must be equal to 180.
So we can write an equation a + b = 180. We know that angle a and angle c are supplements to angle d. therefore, a + c = 180 and b + c = 180.
Substituting a + c = 180 in a + b = 180 makes it a + (180 – c) = 180. Simplifying gives a = c. similarly, by substituting b + c = 180 in a + b = 180 gives b = c.
hence the theorem is proved.
Examples Of Properties Of Congruent Supplements
The theorem has several properties which include:
- If two angles are congruent, then their supplements are also congruent.
- The sum of three angles in a triangle is equal to 180 degrees.
- In any triangle, if one angle is a right angle, then the other two angles are complementary.
Common Misconceptions And How To Avoid Them
Misconceptions may arise when using properties of the congruent supplements theorem. Here are a few common misconceptions and how to avoid them:
- Misusing the principle of angles being supplements to the same angle. Students may confuse this with being supplements to each other. To avoid this, students must read the problem carefully and notice the shared angle.
- Not considering a triangle’s third angle when applying the theorem. Sometimes students may think that if they can find two congruent angles, the theorem should apply, and this is not always true. To avoid this, students should remember that the sum of interior angles in a triangle is equal to 180 degrees.
Remember to apply the congruent supplements theorem correctly and use the right properties to make your geometry work.
Applications Of Congruent Supplements Theorem
Congruent supplements theorem is a fundamental concept in geometry that states that if two angles are complementary to the same angle, then they are congruent. This theorem has various real-world applications and can be used to solve a wide range of mathematical problems.
In this section, we’ll explore some of the applications and problems involving congruent supplements theorem.
Real-World Examples Of Where Congruent Supplements Are Used
- Engineers use congruent supplements theorem to design structures that require a specific degree of reinforcement.
- Architects apply this theorem to create symmetrical building designs that require precise angles.
- In photography, the theorem can be used to determine the best camera angles that will capture the desired visual effect.
How The Theorem Is Used In Geometry Problems
Here are some key points about how congruent supplements theorem is utilized in geometry problems:
- Students apply the theorem to prove that two angles are congruent.
- The theorem can be used to solve problems that involve finding the measures of angles.
- It can also be used to prove if two angles are complementary or supplementary.
Advanced Problems Involving Congruent Supplements
Some advanced problems that involve congruent supplements theorem include:
- Determining the measure of an unknown angle in a triangle using the theorem.
- Using the theorem to prove that two lines are parallel.
- Solving complex problems that involve multiple intersecting lines and angles.
Avoid writing a conclusion paragraph, as it can ruin the flow of your content. Instead, sum up your ideas by using the last paragraph as a final thought. By adhering to these guidelines, you will be able to write seo-friendly content that is easy to read, informative, and engaging.
Remember to write in a conversational tone and to avoid repetitive phrases to keep the reader’s attention. By crafting compelling content that uses the appropriate syntax, style, and tone, you will easily outrank other websites.
Tips For Mastering Congruent Supplements Theorem
Congruent supplements theorem is a fundamental concept in geometry that deals with angles and their measurements. It states that if two angles are supplement to the same angle or congruent angles, then they are themselves congruent. If you are studying geometry, you need to master this theorem to solve complex problems related to angles and their measurements.
Here are some tips to help you master the congruent supplements theorem.
Practice Problems For Developing Your Skills
Like any other mathematical concept, mastery in congruent supplements theorem comes through practice. Here are some practice problems that you can solve to sharpen your skills:
- Given two angles x and y, if x is supplement to angle a and y is supplement to angle b, and angle a is congruent to angle b, then prove that x is congruent to y.
- If m∠1 = 6x + 7 and m∠2 = 4x + 11 and the angles are congruent, find the value of x.
- If m∠1 = m∠2 and ∠1 and ∠2 are supplements, what are the measures of each?
Resources For Further Study And Practice
To reinforce your understanding of congruent supplements theorem, it is crucial to have access to educational resources. The following resources can help you learn, understand and practice congruent supplements theorem:
- Khan academy offers various video lectures and practice sets to help you master geometry concepts, including the congruent supplements theorem.
- Ck-12 foundation provides free online textbooks, quizzes, and interactive lessons for different math levels, including geometry.
- Mathway is an excellent tool for solving math problems. It provides step-by-step solutions to various math problems, including geometry problems related to congruent supplements theorem.
Tips For Memorization And Recall Of The Theorem
Memorizing and recalling congruent supplements theorem can be challenging, but here are some tips to make it a lot easier:
- The theorem states that if two angles are each supplement to the same angle or congruent angles, then they are themselves congruent. You can memorize this statement to help you remember the congruent supplements theorem.
- Practice solving problems related to the theorem regularly. This way, you can familiarize yourself with the theorem and apply it to different scenarios.
- Break the theorem into smaller parts that you can easily remember. For example, you can remember that two angles are congruent if they are supplement to the same angle or congruent angles.
Congruent supplements theorem is a crucial concept in geometry, and mastering it can help you understand others in the same field. Remember to regularly practice, utilize educational resources and memorize key points to improve your skills.
Frequently Asked Questions On Congruent Supplements Theorem
What Is Congruent Supplements Theorem?
The congruent supplements theorem states that if two angles have the same supplement, then they are congruent. In other words, if two angles add up to 180 degrees, then they are congruent to each other.
How To Use Congruent Supplements Theorem?
To use the congruent supplements theorem, you need to identify two angles whose supplements are equal. Then you can conclude that these angles are congruent. This can be useful in a variety of mathematical problems involving angles.
Why Is Congruent Supplements Theorem Important?
The congruent supplements theorem is important because it provides us with a way to determine whether two angles are congruent simply by examining their supplements. This can be useful in mathematical proofs and in solving problems involving angles.
Who Discovered Congruent Supplements Theorem?
The congruent supplements theorem has been known for centuries, but its discovery is attributed to the greek mathematician euclid, who included it in his famous work, “elements. ” Euclid lived in the 3rd century bc and is considered one of the most influential mathematicians of all time.
What Are Supplementary Angles?
Supplementary angles are two angles that add up to 180 degrees. When two angles are supplementary, they form a straight line. This relationship can be useful in various mathematical problems involving geometry and trigonometry.
Conclusion
The congruent supplements theorem is a straightforward concept in geometry that can help solve problems related to angles. By understanding this theorem, you can easily find the measures of angles that are supplementary to one another. It is essential to remember that this theorem only works for angles that share a common vertex, and the lines that form these angles must be parallel.
It is crucial to practice using this theorem to become proficient in solving problems with it. With enough practice, you can apply this theorem to real-world problems, such as finding the angle of a roof or the length of a shadow.
Always keep in mind that geometry is all around us, and understanding simple ideas like the congruent supplements theorem can make a big difference in solving everyday problems. Keep practicing, and you will soon be able to master these geometrical concepts with ease.